Disclaimer: machine translated by DeepL which may contain errors.
New Thermodynamic Laws in Macroscopic Chemical Reaction Systems
Kohei Yoshimura (Department of Physics, 2nd Year Master's Student) |
Sousuke Ito (Universal Biology Institute / Lecturer, Department of Physics) |

Thermodynamics of macroscopic systems has an important place in modern science due to its wide range of applications. In particular, chemical thermodynamics of macroscopic chemical reaction systems plays an important role in determining the direction of chemical reactions. Conventional thermodynamics of macroscopic systems has mostly dealt with transitions between equilibrium states, and the part of thermodynamics that deals with non-equilibrium states away from equilibrium has been considered incomplete. In recent years, however, there has been a growing interest in studying the thermodynamics of non-equilibrium states using small systems in which the effects of "fluctuations," such as Brownian motion and other stochastic noise, are not negligible. Since stochastic description is possible for small systems, fluctuation indices such as dispersion can be introduced, and new thermodynamic laws using such fluctuation indices have been found.
Among the new thermodynamic laws, the thermodynamic uncertainty relation has recently been studied. This equation expresses a trade-off relationship between the fluctuation index and the entropy production rate (equivalent to the rate of decrease of Gibbs free energy in a closed chemical reaction system), where one is larger than the other. It is also known that this relationship provides a time limit for transitions between states. On the other hand, it has not been clear whether such an equation is valid for macroscopic chemical reaction systems in which fluctuations are invisible.
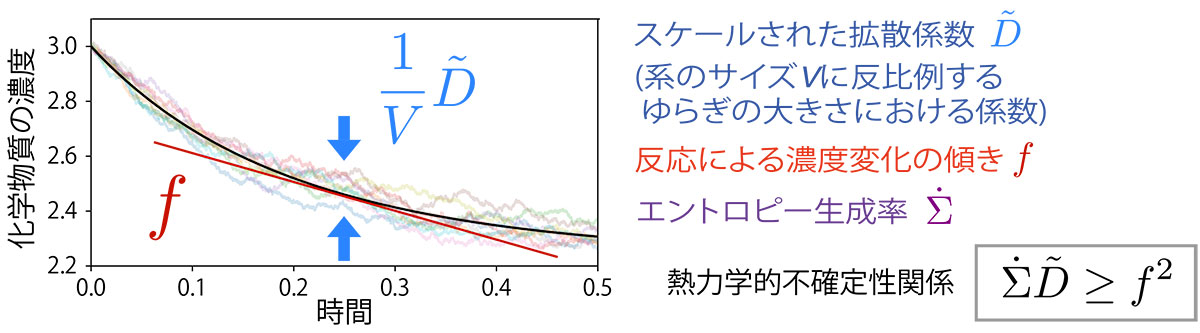
To address this question, we focused on a relation that decreases the magnitude of fluctuations inversely proportional to the size of the system, which corresponds to the reason why fluctuations are invisible in macroscopic systems. While the fluctuations of the system cannot be described by macroscopic quantities, the coefficients in the inversely proportional relationship can be described only by macroscopic quantities. We consider these coefficients as intrinsic fluctuation quantities and call them "scaled diffusion coefficients." Using these coefficients, we have succeeded in presenting an equation similar to the thermodynamic uncertainty relation in the chemical thermodynamics of macroscopic systems. The trade-off relationship between the speed of Gibbs free energy decrease and the "scaled diffusion coefficient" was newly found in a closed macroscopic chemical reaction system.
 |
||
| Figure: When the concentration of a chemical changes in a chemical reaction, there is an intrinsic fluctuation that is inversely proportional to the size V of the system. We have shown that there is a trade-off between the amount of this intrinsic fluctuation (scaled diffusion coefficient) and the entropy production rate. | ||
This result provides a more detailed limit on the rate of chemical reactions than the conventional thermodynamic laws, and has a variety of possible applications. For example, in biochemical reactions where reaction rates are important, it has been believed that there is a trade-off relationship between the accuracy of information processing in vivo and thermodynamic cost.
This research result was published in K. Yoshimura and S. Ito, Phys. Rev. Lett. 127, 1606018 (2021), and was selected as Editors' Suggestion.
(Press release on October 12, 2021)
Published in the January 2022 issue of Faculty of Science News
Communicating to Faculty Research Students on the Frontiers of Research>


