DATE2022.10.12 #Press Releases
Quantum computer calculations of electronic wave packets of nitrogen molecular ions in intense laser fields
Disclaimer: machine translated by DeepL which may contain errors.
Takanori Nishi, Project Researcher, Department of Chemistry
Erik Lötstedt, Associate Professor, Department of Chemistry
Kaoru Yamauchi, Professor, Department of Chemistry
Key Points of the Presentation
- The world's first empirical study of atomic and molecular processes occurring in an intense laser field using an actual quantum computer.
- The electron wave packet of a nitrogen molecular ion driven by an intense laser field is represented by a variational quantum circuit and its time evolution is calculated. The quantum computer ibm_kawasaki was used.
- By applying Clifford data regression (CDR) as an error suppression method, the time evolution of the electron wave packet was successfully reproduced qualitatively.
Summary of Presentation
Simulation of time evolution of quantum systems is one of the most important problems to which quantum computation can be applied. If the simulation of time evolution is performed using the simplest quantum algorithm, the quantum circuit becomes longer as the simulation time increases, and the effect of noise becomes too large for currently available quantum computers, making it difficult to obtain correct calculation results. Therefore, there is an urgent need to provide guidance on the problems of "how to construct efficient and short quantum circuits" and "how to correct errors in the results of calculations using such quantum circuits to predict more correct values.
Associate Professor Lötstedt and Professor Yamauchi and their research group at the Graduate School of Science, The University of Tokyo, have launched the "Applied Quantum Chemistry with Qubit (AQUABIT)" project ( https://www.u-tokyo.ac. jp/adm/fsi/en/projects/quantum/project_00064.html) with the support of DIC Corporation, and has been working on the "development of a methodology for calculating molecular systems using a quantum computer" and "improvement of calculation accuracy by error suppression of calculation results using a quantum computer (Note 1). (Note 1).
As a part of this study, the time evolution of the electron wave packet of a nitrogen molecular ion driven by an intense laser field was calculated by the quantum computer ibm_kawasaki(Note 2). A quantum circuit was constructed using a small number of variational parameters, and the quantum computer was used to calculate the expectation values necessary to construct the equations that the variational parameters must satisfy. It was then shown that time evolution can be qualitatively reproduced by using a special quantum circuit that can be efficiently computed using a classical computer, estimating errors due to noise in the quantum computer, and performing error suppression.
This paper was selected as a Featured Article among the papers to be published in AVS Quantum Science.
Publication details
When nitrogen molecules ( N2 ) are irradiated by an intense pulsed laser pulse of 800 nm wavelength, ionization occurs at a time near the peak of the pulse when the electric field intensity is at its maximum, and nitrogen molecular ions ( N2+ ) are produced. It is known that the electrons in these nitrogen molecular ions are further driven by the electric field in the second half of the pulse to generate a secondary laser light with a wavelength of 391 nm. As a model to explain this phenomenon, we consider three electronic states of the nitrogen molecular ion: X, A, and B. The electrons in the X state as the initial state are also present in the A and B states due to the electric field in the second half of the pulse The time variation of the occupancy of the three states is shown in Figure 1.
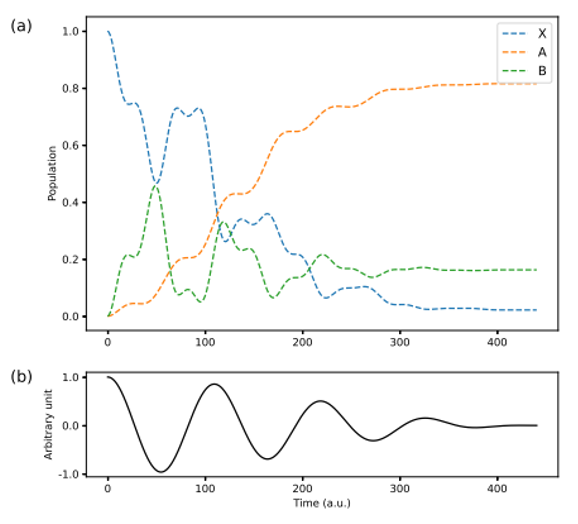
Figure 1: (a) Time variation of the occupancy of the three electronic states of the nitrogen molecular ion, (b) electric field in the second half of the pulse.
Here, (b) shows the electric field in the second half of the pulse. From (a), it can be seen that the occupancy of the X state decreases, and finally the order of the occupancy is A state, B state, and X state, in descending order from the largest occupancy. In particular, the B state is occupied more than the X state, resulting in the emission of a secondary laser light at 391 nm. Such a time evolution is obtained as a solution of the time-dependent Schrödinger equation ![]() . To represent the solution at any given time in a short quantum circuit, a variational quantum circuit
. To represent the solution at any given time in a short quantum circuit, a variational quantum circuit ![]() (Note 3 ) is allowed to act on the initial state
(Note 3 ) is allowed to act on the initial state ![]() , and assuming
, and assuming ![]() , the equation that the time derivative
, the equation that the time derivative ![]() of the variational parameter must follow is given by
of the variational parameter must follow is given by ![]() . M,V is defined as follows.
. M,V is defined as follows.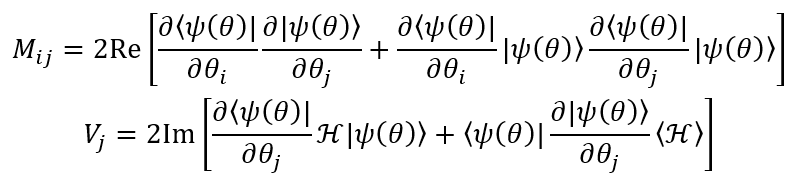
where ![]() is the Hamiltonian. This method of computing M,V on a quantum computer and solving
is the Hamiltonian. This method of computing M,V on a quantum computer and solving ![]() on a classical computer is called the variational quantum simulator method. In this study, we showed that
on a classical computer is called the variational quantum simulator method. In this study, we showed that ![]() can be efficiently approximated
can be efficiently approximated ![]() by expressing by four parameters. To actually calculate M and V in a quantum computer, it is necessary to run a quantum circuit with auxiliary qubits, but when the coupling between qubits is limited, as in ibm_kawasaki, the quantum circuit becomes long, increasing the noise. Therefore, correct calculations cannot be performed without appropriate error suppression. In this study, we applied an error suppression method called CDR.
by expressing by four parameters. To actually calculate M and V in a quantum computer, it is necessary to run a quantum circuit with auxiliary qubits, but when the coupling between qubits is limited, as in ibm_kawasaki, the quantum circuit becomes long, increasing the noise. Therefore, correct calculations cannot be performed without appropriate error suppression. In this study, we applied an error suppression method called CDR.
It is known that two types of quantum gates called Clifford gates and non-Clifford gates (Note 4) are necessary for universal quantum computation. If the number of non-Clifford gates in a quantum circuit is small, the quantum circuit can be efficiently simulated using a classical computer. Therefore, a quantum circuit in which some of the non-Clifford gates of the quantum circuit to be executed are replaced by Clifford gates can be simulated on both a quantum computer and a classical computer, and the results can be compared to estimate the magnitude of the computational error in the calculation on the quantum computer. This estimate can then be used to estimate the magnitude of the computational error in a quantum computer calculation. Based on this estimate, a method to suppress the computational error of a quantum circuit that contains many original non-Clifford gates is called CDR.
Figure 2 shows the simulation results using ibm_kawasaki.
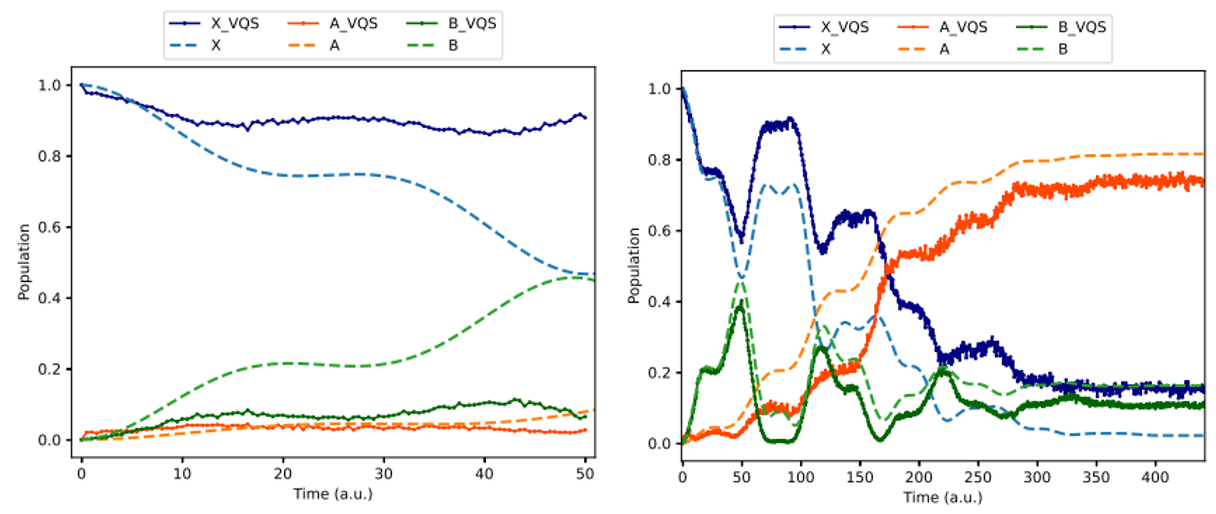
Figure 2: Simulation results using ibm_kawasaki. The exact solution is the dotted line, and the result obtained using ibm_kawasaki is the solid line. On the left is the time evolution of 100 steps calculated without applying CDR, and on the right is the time evolution of 882 steps calculated with CDR.
As shown on the left within Figure 2, when CDR is not applied (solid line), the correct time evolution (dotted line) cannot be reproduced. On the other hand, as shown in the right figure, by applying CDR and suppressing errors in the quantum circuit with auxiliary qubits, the time evolution can be qualitatively reproduced. when applying CDR, there is some freedom in how to choose the Clifford gate that replaces the non-Clifford gate, but in this study, However, in this study, we have shown that CDR can be performed efficiently by employing only the Clifford gates that are most similar to the non-Clifford gates to be replaced, thereby reducing the number of times a quantum computer is used.
Published Journals
-
Journal name AVS Quantum Science Title of paper Simulation of a laser-driven three-level system by a noisy quantum computer Authors Takanori Nishi, Erik Lötstedt, Kaoru Yamanouchi DOI number
Terminology
Note 1 error-suppression
A technique to reduce computational errors in the results of a quantum circuit run. In addition to CDR, which improves results by comparing them with the results of quantum circuits that can be efficiently simulated by a classical computer, a method called zero noise extrapolation is known, in which the relationship between noise and computational error is investigated by changing the magnitude of the noise to estimate the case where the noise is zero. This method is known as zero noise extrapolation. ↑up
Note 2 Quantum computer ibm_kawasaki
A computer that uses a two-level system called a qubit, with the unit of information being a quantum state between |0⟩ and |1⟩. The ibm_kawasaki is a superconducting quantum circuit type quantum computer with 27 qubits, which was introduced to The University of Tokyo in 2021. ↑up
Note 3 variational quantum circuit
A quantum circuit constructed using variational parameters. The type and order of quantum gates used in a quantum circuit are fixed, and quantum computation is performed by optimizing only the variational parameters. In this study, optimization of the variational parameters was performed by solving
. ↑up
Note 4 Quantum gate
A unit of logic operation in a quantum computer, for an element σ of a set S of tensor products of Pauli operators,
for an element σ of the set of tensor products of Pauli operators S is called a Clifford gate, and the others are called non-Clifford gates. ↑up


